From mattang to Konigsberg bridges
- Claudio Carabelli

- 1 dic 2021
- Tempo di lettura: 5 min
The ancient peoples were different from the current one, they explored with acute science knowledge, but in a practical and intuitive way, which were linked to the daily needs of men: it is a real one of the concrete.
With it our ancestors had encountered a knowledge of the endowed with its own organic coherence.
Starting from the 1500s. C. the Polynesians of Fiji, for example, began a series of spectacular journeys that led them to colonize, little by little, all the islands of the Pacific.
An increase in the population and scarcity of food resources, probably the cause at the origin of these migrations.
It must not have been easy to bow their little ones "to the high open sea", in search of tiny islands scattered thousands of miles in the Pacific Ocean.
The Marshall Islands, for example, are an archipelago of 29 atolls, flat, invisible from the sea: how did they manage to identify the coral atolls and return to the "base"?
On their voyages of exploration, the Polynesians were able to predict the presence of an island from minimal clues that testify to an example of what has been called the science of the concrete:
a) smells of flowers: for this they exploited the extraordinary sense of smell of the pigs on board which, once perceived, made them restless
b) observation of large seabirds, such as albatrosses, which generally do not depart more than 40 miles from the coast
c) presence of clouds, which settle more easily on the atolls than on the open sea (the lagoon of an atoll has warmer waters than those of the sea)
d) the observation of currents and waves; when a wave is generated and it encounters an obstacle, the same is reflected: if this obstacle is large, such as an island, behind it a shadow area is generated with waters not disturbed by the waves. Furthermore, if there are more than one obstacles, the reflected waves create crossed ripples on the surface of the sea.
It is precisely by observing these slight modifications of the surface that the Polynesians were able to deduce the presence of an unknown island even before seeing it on the sea.
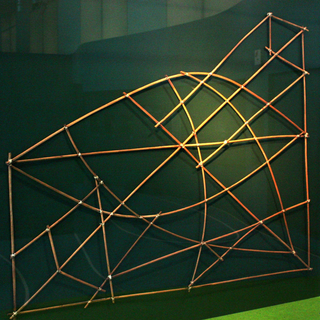
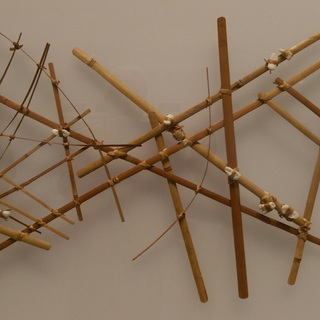
Mattangs were then built to remember and share the position of the islands.
The mattang is an object built with the ribs of palm trees, obtaining a trellis: each stick represented the main currents and winds.
Then other ribs arranged in a V shape were inserted on the trellis, indicating the shaded areas of the currents; finally, shells or pieces of coral were tied to the trellis to position the islands.
The mattang was in effect a real nautical chart, modified and improved with each trip.
Immagini di mattang tratte da Wikipedia
Let's move from Polynesia now to Europe.
What do mattangs have to do with math?
Two answers are possible.
The first report could make the "rude mathematicians" smile, I think it is a bold argument, which will upset someone's neurons, but I propose it anyway: it is a question of correlating these atypical nautical charts to graph theory.
The second answer refers us to none other than Poincaré and the theory of chaos.
The mattangs described above represent a network in which the island shells represent nodes, while the pylons that identify the currents and the shaded areas are segments or arches: from an island, along the right currents, you can reach other islands or return to base.
Well this representation of the ocean space has a structure similar to a graph.
A graph is a mathematical structure made up of elements called nodes or vertices, connected to each other by lines called arcs or edges.
The bridges of Konigsberg
It is said that the inhabitants of Konigsberg (now Kaliningrad, Russian Federation), a town in East Prussia, which became famous because Immanuel Kant was born there, had a problem to face every day.
The town, located on the Pregele river, included two islands: a bridge connected them both; furthermore, the larger island was connected to each shore with another bridge, while the smaller but densely populated one was connected to each shore with two bridges: in total, therefore, there were seven bridges in Konigsberg.
The problem is this: is it possible to find a route that, starting from any area of the city, allows you to cross each bridge once and only once and return to the starting point?

In 1736 it was the famous Swiss mathematician and physicist Leonhard Euler who was the first to find the solution to this question and proved, using graph theory, that the hypothesized walk was not possible.

Euler faced the problem by associating a graph to the city map (see above) and identifying each area with a point (node or vertex) and each bridge with an arc or segment.
In this schematization, the number of arcs or segments exiting a node is defined as the degree or order of the node.
Euler came to formulate laws to solve this type of problem: a graph composed only of even nodes, that is, each connected with an even number of arcs or segments, is always passable and one can always return to the starting point.
Such a graph is called Eulerian and the path is called Eulerian circuit.
If a graph contains even nodes and only two odd nodes it is still viable, starting from one of the odd nodes to get to the other, but it is no longer possible to return to the starting point. Such a graph is not Eulerian and the path is called the Eulerian path.
If, on the other hand, it contains more than two odd nodes, it cannot be covered without path overlaps.
Analyzing the situation at Konigsberg, it can be seen that three bridges depart (and arrive) from nodes C, B and D; from node A, on the other hand, five bridges.
These are the degrees of the nodes: respectively, 3, 3, 3, 5.
It has as many as four odd-order nodes, therefore it is not practicable without overlapping.
The theory of graphs has had an application in various fields in the following centuries:

The relationship between mattang and chaos theory is more convincing

Rocker canoes of the Marshall Islands
The content of a stick chart, such as a mattang, is seen from a new perspective when one compares the physical mechanisms responsible for making atoms and atolls visible.
Wave diffraction due to islands or atolls can be illustrated today by analog physics as neutron diffraction due to particles (Paul Scherrer Institut 2009).
Both mechanisms make visible atolls and invisible atoms.
Using the experimental method of neutron diffraction it is possible to make atoms visible, for example in materials research.
A neutron can also behave like a wave.
The interaction of neutrons with atoms is analogous to the interaction of wave motion with the atolls of the Marshall Islands.
Because of such interactions, interference patterns arise.
From the models of neutron interference, conclusions can be drawn about the structure and movement of atoms.
Similarly to neutron diffraction, the inhabitants of the Marshall Islands could find the position of an invisible atoll by following the nodes (okar) of the overlaps of the wave motion, observing them aboard their outrigger canoes.
It was Henri Poincaré, in an article published in Acta Mathematica in 1890, who faced a similar theoretical situation with a geometric approach.
If one tries to visualize the pattern formed by these two curves and their infinite number of intersections (...), these intersections form a kind of lattice, an intertwining, a network of infinitely fine meshes.It was clear to Poincaré that he had discovered a fundamentally new aspect of dynamic movement.
This was the original discovery of what is now known as initial condition sensitivity, which is at the heart of chaos theory.
Some images taken by satellites demonstrate the evidence of the theory.

Ps: this pragmatism of the Polynesians was mixed with legends to explain some phenomena, such as for example the tides. They therefore resorted to myth to make sense of a phenomenon with which they were in contact every moment.
The theme "From myth to the science of the concrete" can find its didactic position at the beginning of the year in the course of Science of the Lower Secondary School.

Bibliography
L'uomo dalla natura alla scienza, 1993 Giulio Mezzetti La Nuova Italia
La matematica dei social network. Una introduzione alla teoria dei grafi, 2012 Peter M. Higgins Dedalo edizioni
Sitography in the text